DEPARTMENT OF MANAGEMENT SCIENCE
MSCI212: STATISTICAL METHODS FOR BUSINESS
商业统计方法代写 These questions are designed to help you master the lecture material from weeks 3 and 4, i.e. based on chapters 13-16 of Stine…
Week 5 Workshop 商业统计方法代写
These questions are designed to help you master the lecture material from weeks 3 and 4, i.e. based on chapters 13-16 of Stine & Foster. If you find these difficult you may also find some of the questions in the text itself useful.
The statistical ‘tools’ covered in these chapters are essentially to be used for calculating confidence intervals when estimating population means, and for testing hypotheses about population means. These examples require you to decide which tool to use, and to carry out the necessary calculations.
Questions 1 & 2 are based on chapter 13 & 14, questions 3-6 are on confidence intervals (based on chapter 15), and questions 7-13 are on hypothesis testing (based on chapter 16).
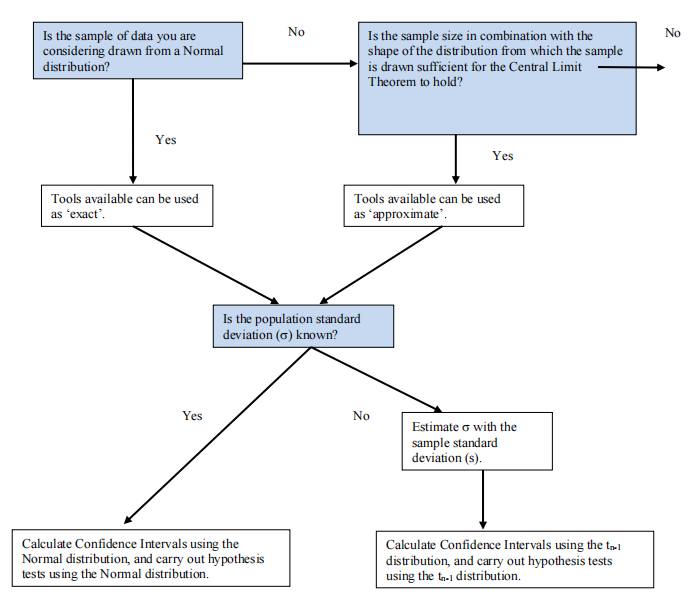
AT your workshop you will work in groups to decide which tool is appropriate for questions 3, 5, 9 and 11, and why. (You may find the decision making diagram on the last page of this worksheet helpful.) You will also work out the answer to one (or more) of these four questions. You should prepare for this BEFORE your workshop.
Other questions are designed for further practice of these skills, and of related skills (e.g. use of finite population correction, choosing sample size, setting hypotheses, using p-values and calculating the power of a test). At the workshop you can ask for help on any of these, and some notes on how to solve them will also be available.
Q1. An accounting firm has a large pool of secretaries. It is assumed that the time it takes a secretary to type a certain legal document has a Normal distribution with a mean of 30 minutes and a standard deviation of 4 minutes. 商业统计方法代写
(a) What is the probability that a secretary will spend less than 27 minutes typing the document?
(b) In a randomly selected sample of six secretaries, what is the probability that the average time it takes them to type the legal document is less than 27 minutes?
Q2. The research and development department of a large oil company employs 253 engineers who have an average of 6.2 years of practical experience with a standard deviation of 2.1 years.
(a) If a sample of 35 engineers is selected randomly without replacement, what is the probability that the average number of years of experience of the sample will be greater than 6.8 years?
(b) If a sample of 35 engineers is selected randomly with replacement, what is the probability that the average number of years of experience will be greater than 6.8 years?
Q3. A quality assurance inspector believes that the weight of a machine bearing produced by a certain manufacturing process approximately follows a uniform distribution. A sample of 50 machine bearings yielded a sample mean of 0.211 pounds. The standard deviation of the Uniform distribution is known to be 0.05 pounds. Find the 90% confidence interval for the mean weight of the bearings produced by this manufacturing process.
Q4. The tensile strength of a high-powered copper coil used in giant power transformers is believed to follow a Normal distribution. A sample of 14 high-powered copper coils yields the following tensile strength (in units of thousands of pounds per square inch). Construct a 90% confidence interval for the mean tensile strength of copper coils. 商业统计方法代写
6.1, 2.6, 3.5, 4.3, 3.1, 5.2, 3.6, 3.5, 5.4, 4.2, 3.2, 2.8, 4.0, 3.7
Q5. A Cadillac dealer wishes to determine if the advertised miles per gallon (mpg) of her new Cadillac is equal to 27mpg. A road test of 15 randomly selected Cadillacs yielded the following results.
{26, 26.5, 23.5, 26, 28, 27.5, 27, 26.8, 27.5 26.8, 25, 27.5, 26.8, 26, 27}
(a) Find a 95% confidence interval for the mean miles per gallon of the new Cadillacs.
(b) Interpret the confidence interval in part (a) for the Cadillac dealer. What assumption is necessary about the distribution of the data?
Q6. Cereal companies like Kellogg view Europe and Asia as an opportunity for growth at a time when the US market is in a decline. In Italy, for example, American cereals like Cornflakes have been available since the 1950s. But until the 1990s, they were considered a niche product. Suppose that Kellogg wishes to estimate the annual consumption by adults in Italy. How large would the sample size need to be to estimate the mean annual consumption by adults (in pounds) to within 0.2 pounds with 95% confidence if the standard deviation of the amount of cereal consumed is estimated to be 1.2 pounds?
Q7. Set up the null hypothesis and the alternative hypothesis for each of the following situations. 商业统计方法代写
- a) An automotive analyst believes that the miles per gallon on a new model is less than what the company is advertising. A random sample of data is taken to look for support for the analyst’s belief.
- b) A marketing research firm believes that the average number of hours per week that Americans watch television differs from the 15 hours a week that it has been in the past. A sample of data is taken to investigate this belief.
- c) A telecommunications monitoring organisation claims that the average customer of a particular company pays at least $30 a month. The company collect a random sample of data to attempt to refute this claim.
Q8. While interest rates on deposits at banks have been relatively low in 1994, the national average interest rate charged by credit card issuers is 17.80%. Suppose that a banker in Chicago believes that most of the credit cards issued by local banks and financial institutions based in Chicago have interest rates lower than the national average. A random sample of 50 credit card issuers revealed that the sample mean of the interest rates was 16.95%. The population standard deviation is known to be 2.2%. What managerial conclusion can you give to the banker, assuming a .01 significance level? Under what conditions is your analysis valid?
Q9. Green Line, a major discount stock brokerage in Canada, claims that customers calling in to make trades on the stock market are left on hold for an average of about 22 seconds. Suppose that an account executive was concerned about the satisfaction level of the clients and selected a random sample of 100 traders who phoned. The length of time that a client was left on hold was recorded. The sample mean was 23.5 seconds. The population standard deviation is known to be 8 seconds. Is there sufficient evidence to indicate that customers are left on hold for an average length of time greater than 22 seconds? Use a .05 significance level. Under what conditions is your analysis valid?
Q10. Many businesses are increasingly concerned with employment equality programs that attempt to integrate more women and minorities into the ranks of technical and managerial positions. A Canadian survey of the major industries in Canada indicated that, on average, a medium-to-large firm had 14 women in senior management positions. Suppose that the president of an organisation for the advancement of women in Canadian businesses believed that the mean number of women in senior management positions within medium-to-large energy-related firms is less than 14. Assume that the number of women in senior management positions at medium-to-large Canadian firms is approximately normally distributed with a standard deviation of 5. 商业统计方法代写
What is the power of the hypothesis test to test the president’s belief, if the sample size for a random sample of medium-to-large Canadian firms was 35 and if the true mean number of women in senior management positions at this type of firm was 12? Use a .05 significance level. Under what conditions is your analysis valid?
Q11. In December 1993, Business Week magazine claimed that the average yield on an interest-bearing account was 1.65%. A bank depositor wishes to know if the yield on interest-bearing accounts for banks in Delaware was different from the national average. The bank depositor believes that the yields on interest bearing accounts are approximately Normally distributed. A random sample of 25 banks yielded a sample mean of 1.78% with a standard deviation of .25%. Is there sufficient evidence to indicate that the yield on interest-bearing accounts for banks in Delaware is different from the national average? Use a .05 significance level. Under what conditions is your analysis valid?
Q12. The senior executive of a publishing firm would like to train employees to read faster than 1000 words per minute. A random sample of 21 employees underwent a special speed-reading course. This sample yielded a mean of 1018 words per minute with a standard deviation of 30 words per minute. Does the data support the belief that the speed-reading course will enable the employees to read more than 1000 words per minute at a significance level of .05? Under what conditions is your analysis valid? 商业统计方法代写
Q13. A manufacturer of drugs and medical products claims that a new anti-inflammatory drug will be effective for 4 hours after the drug is administered in the prescribed dosage. A random sample of 50 volunteers demonstrated that the average time is 3.70 hours. The population standard deviation is known to be 0.606 hours. Use the p-value criteria to determine if there is sufficient evidence to support the hypothesis that the mean effective time of the drug differs from 4 hours. Under what conditions is your analysis valid?
Choosing the correct statistical ‘tool’ when calculating confidence intervals or testing hypotheses about population means.