Task #2 Leontief Model
线性代数代写 Leontief Model was first proposed by the Nobel Prize winner Wassily Leontief, as is told in the first page of our textbook.
[Subject: Industrial Organization] 线性代数代写
Leontief Model was first proposed by the Nobel Prize winner Wassily Leontief, as is told in the first page of our textbook. It is also known as input-output (IO) model. An IO model is constructed from observed data for a particular economic area – a nation, a region (however defined), a state, etc. The economic activity in the area must be able to be separated into a number of segments or producing sectors, which may be industries in the usual sense. You may find more information on Page 49, Section 2.6 in the textbook.
The necessary data are the flows of products from each of the sectors (as a producer/seller) to each of the sectors (as a purchaser/buyer), or, for example, from Sector to Sector . These transaction data are usually designated as a matrix , whose elements are , recording the monetary values of the transactions between such pairs of sectors. Finally, all products are sold to some exogenous units such as households, government, and foreign trade. Scholars usually call them final demand outside the IO model. 线性代数代写
Now assume that the economy can be categorized into sectors. If we denote by the total output of sector and by the total final demand for sector ‘s product, we may write a simple equation accounting for the way in which sector distributes its product through sales to other sectors and to final demand:
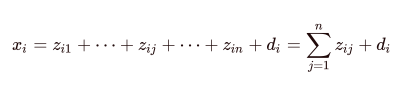
The Zij terms represent interindustry sales by sector (also known as intermediate sales) to all other sectors. According to the above information, you are required to complete the following questions: 线性代数代写

- Using matrices (including ) and vectors to represent Eq.( ) for all sectors. What kind of information does the th column of tell us?
- Given and , for example, input of aluminum ( i) bought by aircraft producers (j ) last year and total aircraft production last year, form the ratio of aluminum input to aircraft output, Zij/Xj , and denote it by , or known as technical coefficient.
(a) Now replace your answer in the previous exercise using a matrix whose elements are given by . What is the relationship between and .(b) Show that, where is a diagonal matrix with the elements of the vector along the main diagonal.
- Consider a hypothesis case that Using MATLAB to compute the final demand for all . 线性代数代写
- Go to National Bureau of Statistics of China (NBS) and download the 2012, 2015 and 2017 version IO Table of China.
(1) Try to generate three technical coefficient matrices in MATLAB.
(2) Choose one particular industry and analyze how one unit change of final demand on its product will affect the total output of this industry. Repeat this analysis for all three years.




