MATH 153 E
MATH序列代考 The exam is out of 150 points. Partial credits will be given. You should give reasons or show your work.
- The exam is out of 150 points.
- Partial credits will be given.
- You should give reasons or show your work.
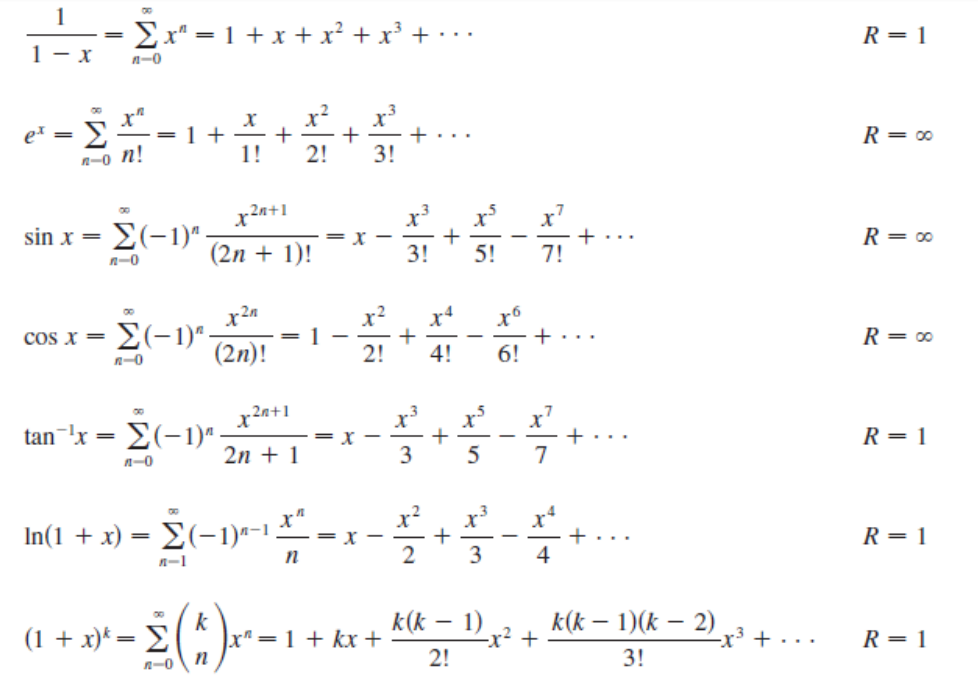
- (4p.) Find a formula for the general term an of the sequence, assuming that the pattern of the first few terms continues:
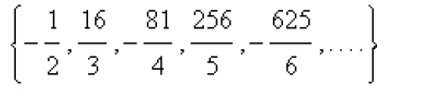
- (12p.) Determine whether the sequence converges or diverges. If it converges, find the limit. MATH序列代考

- (12p.) Determine whether the series is convergent or divergent. If it is convergent, find its sum.

- (10p.) Use the binomial series to expand the function as a power series. State the radius of convergence: (1+ x)3/4 .
-
(32p.) Test the series for convergence or divergence. MATH序列代考
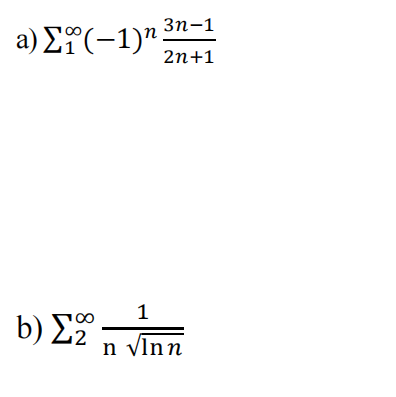
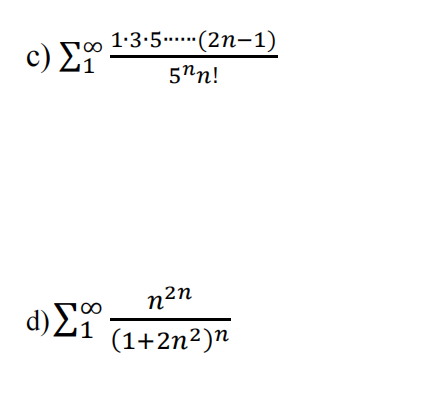
- (8p.) Use any test to determine whether the series is absolutely convergent, conditionally convergent, or divergent.
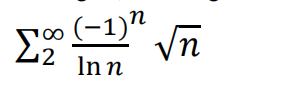
-
(8p.) Find the radius of convergence and interval of convergence of the series: MATH序列代考
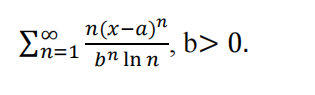
- (10p.) Express the function as the sum of a power series by first using partial fractions. Find the radius of convergence. f(x) =(2x – 4)/(x2 – 4x + 3).
- (8p.) Show that 0.9999…= 1
- (10p.) Find the Maclaurin series for f (x) using the definition of a Maclaurin series. Also find the associated radius of convergence: f(x) = 10x
- (12p.) Find the Taylor series for f (x) centered at a = π/6 using the definition of a Taylor series ???? ???? ?ℎ? ?????????? ?????? ?? ???????????: ?(?) = ????.
-
(8p.) Use series to evaluate the limit. MATH序列代考
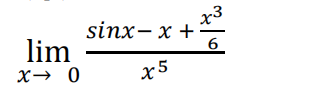
- (8p.) Find the sum of the series.
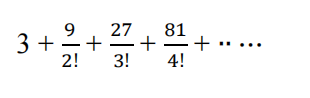
- (8p.) Evaluate the indefinite integral as a power series. What is the radius of convergence?
∫ ?² ln(1 + ?) ??




