Practice Exercise, on pricing and arbitrage
Mathematical Finance代写 I discovered that there is quite a number of solved exercises (and some unsolved ones) in the book…
I discovered that there is quite a number of solved exercises (and some unsolved ones) in the book Mathematical Finance, Theory Review and Exercises. From Binomial Model to Risk Measures, by Rosazza Gianin, Sgarra. Which is can be consulted online via Imperial’s library going at this address. The difficulty of these exercises is appropriate.
Watch out, these authors often (always?) write the final wealth using the variables which I called (h0 , h1), instead of those which I called (x, h). However, they do not use the (h0 , h1) notation; rather, they write (x, ∆); in particular what I called x is the initial capital. Whereas what they call x is the amount of money deposited in the bank account at time 0.
For exercises on the topics we covered in our class, see: Mathematical Finance代写
- exercises on pricing in the multi-period binomial model: chapter 3
- exercises on absence of arbitrage and completeness of markets: chapter 4 (skip Exercise 4.5 item 4)
- exercises on pricing if exotic options in the binomial model: exercises 9.4, 9.5
For you to understand these exercises and solutions, I need to clarify a few points:
- Their ’risk-free interest rate’ is what we simply called ’interest rate’. When they say ’the non-risky asset’ or ’the bond’, they mean what we normally called ’the bank account’.

3. Exercise 9.4 considers a Lookback Call option on the underlying S = (St)Tt=0; this is defined as having payoff ST − MT , where the process Mu := mint=0,1,…,u St is the running minimum of S. Btw, this exercise mentions ’(without dividends)’; please just ignore this (stocks can pay dividends. Which leads to some uninteresting complications, so we did not cover this in class).

- In the provided solution to exercise 3.2 item 3, at pages 42 and 43, there appears a table describing the P&L (Profit or Loss) of the strategy, in which for the stock there is written ST − S0 (payoff minus cost), whereas for the other entries (calls, put, bank) there only appears their payoff. This is wrong: the P&L should always be always payoff minus cost, not just for the stock, but also for the bank account and also for the options. So, in the first setting (if the stock changes value in 3 months), the P&L is (rounding to the 2 decimals):
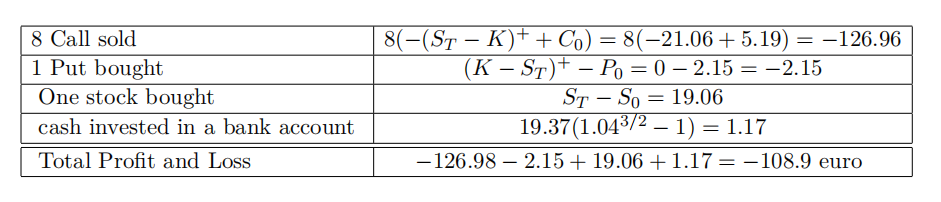
Since the sum of the initial costs is zero (= the initial investment), of course to calculate the total P&L, one could alternatively write only the payoffs instead of payoff minus cost, i.e. write: Mathematical Finance代写
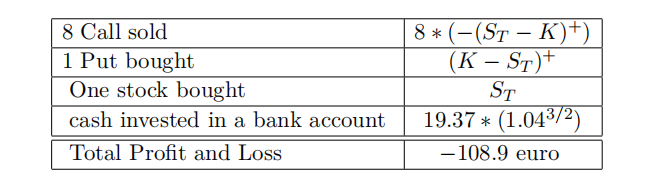
This solution is very similar to what’s written in the book. As it only differs in the term relative to stocks (where ST appears, instead of ST − S0). However, the first solution seems preferable, since it provides also the P&L for each individual investment, not just the total one.




