BEEM101
Mid-term Exam
MICROECONOMICS
微观经济学mid代考 To achieve full points for a problem it is not sufficient to provide the correct final answer. I must be able to retrace every…
Duration: 1 hour + 30 minutes upload time
No word count specified
This is an Open Book
To achieve full points for a problem it is not sufficient to provide the correct final answer. I must be able to retrace every step that took you to your answer.
-
Problem – Preferences (24 points) 微观经济学mid代考
Luca’s preference relation ≽ on the set of alternatives X = {mango,kiwi,pineapple,banana} fulfills mango≽kiwi, pineapple≽mango, and kiwi≽banana.
a) What can you conclude from completeness of ≽ about Luca’s preferences for pineapple compared to kiwi?
b) What can you conclude from transitivity of ≽ about Luca’s preferences for pineapple compared to kiwi?
c) What can you conclude from transitivity of ≽ about Luca’s preferences for banana compared to pineapple?
d) Suppose that in addition to the information given above, you learn that Luca’s preferences satisfy mango≻kiwi. Show that now pineapple≻kiwi is implied.
-
Problem – Utility (16 points)
Consider the set of alternatives containing all integers from -5 to 5 except 0, i.e. X = {−5, −4, …, −1, 1, …, 5}. Lisa’s preference relation ≽ on the set X is represented by the utility function u(x) =1x .
a) In your own words, describe what it means that the above utility function represents Lisa’s preferences.
b) Give an example of another utility function that represents Lisa’s preferences. Explain your answer.
c) Give an example of a function that does not represent Lisa’s preferences. Explain your answer.
3.Problem – Utility (7 points) 微观经济学mid代考
In the lecture, we stated the following theorem: “Every preference relation (complete, reflexive, transitive) on a finite set of alternatives can be represented by a utility function.” Briefly discuss what this statement means and why it is important.
Note: Copying what is written on the slides is not sufficient to achieve full points for this question.
-
Problem – Choice (6 points)
Consider the set of alternatives X = {a, b, c}. Give an example of a choice function for X.
-
Problem – Choice (23 points)
Suppose you observe the following data about Linda’s coffee choices on campus:

Note: Assume that according to Linda’s preferences the best alternative in any given choice set is unique.
a) Could Linda’s choices in principle be rationalizable? If your answer is yes, give an example of a preference relation that can accommodate her choices. If your answer is no, explain.
b) From the information given, can you determine whether Linda’s choice function satisfies Property α? Explain your answer.
c) Suppose you make the following additional observation of a coffee choice by Linda:
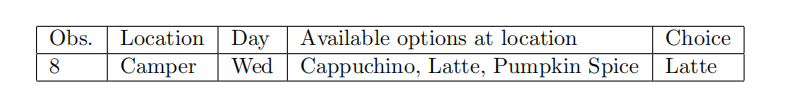
Does this observation change your answer to part a)? Explain.
d) Take a step back and think about the answer that you gave in part c) of this problem. Are there any additional assumptions about Linda’s preferences or choice problems that you implicitly(or explicitly) made to arrive at your conclusion? If your answer is yes, name one and explain why it is needed. If your answer is no, explain.
-
Problem – Consumer preferences (24 points) 微观经济学mid代考
Let X = R 2 + be the set of all bundles of lemons and limes. Denote by x1 the amount of lemons and by x2 the amount of limes contained in bundle (x1, x2) ∈ X. For each of the following individuals, give an example of a utility function that represents their preferences, calculate the corresponding marginal rate of substitution for lemons in units of limes (where possible), and sketch the corresponding indifference curve map.
Note: In your drawings, make sure to include axis labels and minimal grid information such that the most important characteristics of the indifference curve map like slope, vertical/horizontal intercept, direction of improvement, and the location of important points can be identified.
a) Lara likes the juice of lemons just as much as she likes the juice of limes. Lara can get three times more juice out of one lemon than out of one lime.
b) Laurence dislikes anything sour. To Laurence, a lemon tastes four-times more sour than a lime.
c) Lucy is a big fan of the cocktail Caipirinha. To prepare Caipirinhas she only needs limes which is why she does not care for lemons at all.
d) Leo uses lemons and limes only for decorative purposes. To create a balanced color palette, he insists that the only proper way of combining lemons and limes in a fruit bowl is to have two limes for every lemon in the bowl.




